Geometric Performance Attribution
Return attribution is a technique that is used to identify and quantify the performance of an actively managed fund versus its benchmark. The most popular methods are the Brinson-Fachler (Brinson model) and the factor based attribution approach. Generally, these approaches are designed to explain the excess return, that is the portfolio return minus the benchmark return. The result is an arithmetic attribution.
The geometric performance attribution approach improves upon the arithmetic approach by classing the geometric excess return. On this page we discuss the geometric performance attribution formula and illustrate the approach using a numerical example. The spreadsheet can be downloaded at the bottom of the page.
Geometric performance attribution formula
As we discussed in the introduction, the arithmetic return is designed to explain any excess return earned by the portfolio (R) and that of its appropriate benchmark (B). Geometric attribution improves upon the arithmetic approach by classifying the geometric excess return (G)
The geometric excess return (G) is equal to the ratio of the arithmetic excess return (R-B) over the wealth ratio of the portfolio’s appropriate benchmark (1+B).
No smoothing approaches are needed to adjust the geometric attribution for affects over multiple periods, which is the case when arithmetic attribution is used.
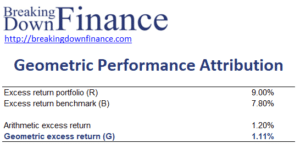
Example
Let’s consider a simple numerical example of how to apply the above formula. The following table converts the arithmetic attribution return into a geometric attribution return
The spreadsheet can be downloaded at the bottom of the page.
Summary
To summarize, arithmetic attribution is best suited for use with nontechnical clients and in marketing reports, whereas geometric attribution is best suited for use with industry. In most cases, the arithmetic attribution approach is used, while geometric attribution is rarely used in practice.
Download the Excel spreadsheet
Want to have an implementation in Excel? Download the Excel file: Geometric Performance Attribution Example