Target portfolio beta
Futures contracts on equity indices can be used to decrease the beta of a portfolio by shorting them, and long positions can be used to increase the beta of a portfolio. A portfolio’s beta equals the weighted average of the betas of the individual stocks in the portfolio. On this page, we discuss how to calculate the hedge ratio, i.e. the necessary number of contracts to change the beta of an existing portfolio to a certain target. The target portfolio beta is often changed dynamically by global macro hedge funds.
A spreadsheet that implements the approach is available for download at the bottom of the page.
Target portfolio beta formula
The number of contracts needed to change the beta of an existing portfolio can be calculated using the following formula
where betaT is the target beta, betaP is the current portfolio beta, betaF is the futures beta (beta of the stock index), MVP is the market value of the portfolio, and F is the futures contract value (i.e. futures price times multiplier).
If we wish to fully hedge the portfolio, then the target beta equals 0.
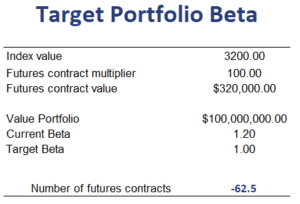
Target portfolio beta example
Next, let’s consider a simple numerical example that implements the above concepts. The following table shows the results of a fund manager that wishes to achieve a target beta using index futures. Notice that the beta of the futures contract is 1 by definition
Note that if the number of required contracts is negative, then we have to sell futures contracts. The Excel spreadsheet can be downloaded at the bottom of this page.
Summary
We discussed how fund managers can alter their portfolio beta using a target beta and futures contracts.
Download the Excel spreadsheet
Want to have an implementation in Excel? Download the Excel file: Target Beta calculator