Projected Benefit Obligation (PBO)
The Projected Benefit Obligation (PBO) or present value of defined benefit obligation (PVDBO) is the actuarial present value of all future pension benefits that are earned by the employees to date. It is based on expected future salary increases. Calculation of the PBO assumes the company is a going concern and that employees will stay with the company until retirement.
On this page, we discuss the components that change the PBO from year to year. Will also discuss the periodic pension cost. This is not the same as the PBO. In particular, the periodic pension cost measures the employer’s contributions adjusted for changes in the funded status.
Projected benefit obligation definition
The change in the PBO is the result of the current service cost, the interest cost, past (prior) service cost, changes in the actuarial assumptions and benefits paid to employees. Let’s discuss each of these components in more detail.
- Current service cost: the present value of the benefits earned by the employees over the fiscal year
- Interest cost: the increase in the obligation due to the passage of time
- Past (prior) service cost: retroactive benefits awarded to employees
- Changes in actuarial assumptions: gains and losses that results from changes in the discount rate, mortality, employee turnover
- Benefits paid: benefits paid to employees that lower the obligation
Together, these components make up the change in the PBO. Let’s translate this into a formula.
Projected benefit obligation formula
The PBO formula looks as follows:
We can use the PBO to calculate a plan’s funded status. Using the funded status formula, we obtain
Let’s also have a look at the definition for the total periodic pension cost (TPPC). It is important to keep in mind that this is NOT the same as the change in the PBO. The periodic pension cost formula equals
Finally, let’s have a look at an example.
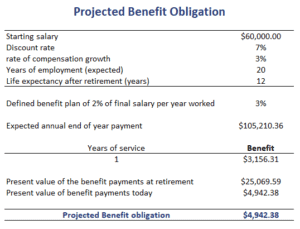
Projected benefit obligation example
The following table illustrates the necessary calculations to determine the change in the PBO. Clearly, a simple spreadsheet is sufficient to perform the necessary calculations. The spreadsheet we used as an example is available for download at the bottom of the page.
Summary
We discuss the PBO, and how to calculate the annual change in the PBO. This is a very important measure to calculate when analyzing a company that has a defined benefits pension plan.
Download the Excel spreadsheet
Want to have an implementation in Excel? Download the Excel file: Projected Benefit Obligation template