Duration-based hedge ratio (BPVHR)

The duration-based hedge ratio (BPVHR) is used to hedge interest rate risk of long-only bond portfolios. To hedge the risk, the fund manager will sell Treasury bond futures. As interest rates rise, bond prices fall and the future price decreases, increasing the value of a short futures position. 

On this page, we explain how fund managers use fixed income futures to hedge interest rate risk, explain how to hedge basis or spread risk, and discuss the duration-based hedge ratio (BPVHR) formula. We also include an Excel spreadsheet that implements the BPVHR formula.

Hedging interest rate risk

Futures contracts are typically used by portfolio managers to achieve a target duration. They can be used to reduce duration (by shorting futures) or increase duration (by buying futures). When hedging interest rate risk, the first step is to identify the futures contract CTD bond. We discuss how to do this on the page on the cheapest-to-deliver bond.

Treasury futures will correlate most with the CTD bond because that bond has the lowest basis of any deliverable bond. As a consequence, the change in the futures price will equal the change in the value of the CTD adjusted by its CF (i.e., the CTD and futures contract have the same duration)

    $$ \Delta \textrm{futures price} = \frac{\Delta CTD}{CF} $$

To fully hedge the portfolio’s value against interest rate changes, the change in the portfolio value must be offset by the change in the futures value

    $$ \Delta \textrm{P} = \frac{HR}{\textrm{\Delta futures price}} $$

where HR is the hedge ratio (number of futures contracts) and Delta P is the change in price. We can rewrite the formula as follows

    $$ \textrm{HR} = \frac{\Delta P}{\Delta \textrm{CTD}} \cdot CF $$

Basis risk

In practice, the CTD bond and the portfolio the investor wishes to hedge are unlikely to be perfect substitutes. The mismatch between changes in the value of an asset or portfolio and the change in value of the derivative used to hedge is referred to as spread risk or basis risk.

BPVHR formula

If the portfolio does not consist solely of the CTD bond, then we use the BPVHR. It calculates the number of futures contracts requireds for a hedge:

    $$ \textrm{BPVHR} = \frac{-BPV_{portfolio}}{BPV_{CTD}} \cdot CF $$

Where BPV is the basis point value. It equals the expected change in value of a security or portfolio given a one basis point (0.01%) change in yield. BPVHR is the number of short futures. We implement the above formula in more detail in the following example, which is available for download at the bottom of the page.

BPVHR example

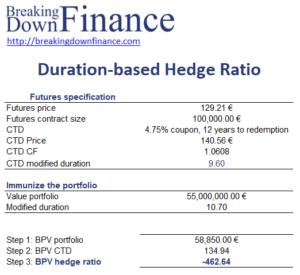
Let’s consider a numerical example that illustrates how to calculate the duration-based hedge ratio using an Excel spreadsheet. The following figure shows the calculations. The spreadsheet can be downloaded at the bottom of this page.

Duration-based Hedge Ratio

Summary

We discussed how to hedge a bond portfolio using treasury futures. In particular, the fund manager will short Treasury future contracts. To determine the number of futures, we calculate the hedge ratio.